




Next: Linear Dissipation (`Ohmic Bath')
Up: `Semiclassical' Limit for Damped
Previous: Wigner Distribution in `Semi-classical'
Contents
Index
In the semi-classical approximation, the time-evolution of the Wigner-distribution function
is determined by Eq. (7.249). This equation describes
a stochastic process for the center-of-mass co-ordinate 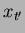 of the
particle, moving within a potential
of the
particle, moving within a potential 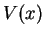 under the action of a
deterministic friction force
under the action of a
deterministic friction force
![$ F_B[x_s,t']$](img1002.png) ,
,
![$\displaystyle F_B[x_s,t'] = -\sum_{\alpha=1}^N \int_{0}^{t'}ds
\frac{\sin \Omega_\alpha (t'-s)} {M_\alpha\Omega_\alpha} f'_\alpha[x_{t'}]f_\alpha[x_{s}],$](img1052.png) |
|
|
(246) |
and a stochastic force
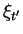 . The motion of the particle is governed by the stochastic integro-differential
equation
. The motion of the particle is governed by the stochastic integro-differential
equation
![$\displaystyle M\ddot{x}_{t'} + V'(x_{t'}) + F_B[x_s,t'] =\xi_{t'},$](img1054.png) |
|
|
(247) |
where the stochastic force 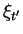 is a random force which itself depends
on the coordinate of the particle: this can be seen by the fact that its variance,
is a random force which itself depends
on the coordinate of the particle: this can be seen by the fact that its variance,
![$\displaystyle \langle \xi_{t'} \xi_{s} \rangle = \varphi_2[x]= \sum_{\alpha=1}^...
...a\Omega_\alpha}{2}
\cos \Omega_\alpha (t'-s) f'_\alpha[x_{t'}]f'_\alpha[x_{s}],$](img1055.png) |
|
|
(248) |
depends on the particle path 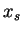 . The Wigner distribution is obtained by
integrating over all possible realisations of the stochastic force, such that
Eq. (7.252) is fulfilled.
Since the
. The Wigner distribution is obtained by
integrating over all possible realisations of the stochastic force, such that
Eq. (7.252) is fulfilled.
Since the 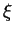 path-integral is Gaussian, one speaks of a Gaussian stochastic
process. This of course is due to the fact that we truncated the
path-integral is Gaussian, one speaks of a Gaussian stochastic
process. This of course is due to the fact that we truncated the 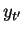 expansion in the
influence phase after the term quadratic in
expansion in the
influence phase after the term quadratic in 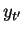 .
.
Our results shows that the influence of the bath is two-fold: it leads to a deterministic,
retarded `friction' force, and to a stochastic force. The latter contains the
temperature and, by means of the
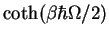 terms in
terms in
![$ \varphi_2[x]$](img1058.png) , a fully quantum mechanical description of the bath.
, a fully quantum mechanical description of the bath.
For certain types of system-bath couplings, one can explicitely show that the operator
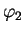 is positive definite and therefore, the term quadratic in
is positive definite and therefore, the term quadratic in 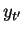 in the original double path integral
exponentially suppresses strong deviations from the diagonal paths with
in the original double path integral
exponentially suppresses strong deviations from the diagonal paths with 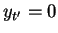 .
In this case, the expansion of the one-particle potential
.
In this case, the expansion of the one-particle potential 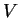 ,
,
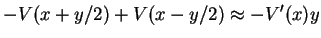 |
|
|
(249) |
becomes more plausible. One should, however, expect that quantum mechanical effects
(like particle tunneling) are destroyed by the approximation Eq. (7.253).





Next: Linear Dissipation (`Ohmic Bath')
Up: `Semiclassical' Limit for Damped
Previous: Wigner Distribution in `Semi-classical'
Contents
Index
Tobias Brandes
2004-02-18
![$\displaystyle F_B[x_s,t'] = -\sum_{\alpha=1}^N \int_{0}^{t'}ds
\frac{\sin \Omega_\alpha (t'-s)} {M_\alpha\Omega_\alpha} f'_\alpha[x_{t'}]f_\alpha[x_{s}],$](img1052.png)
![$\displaystyle F_B[x_s,t'] = -\sum_{\alpha=1}^N \int_{0}^{t'}ds
\frac{\sin \Omega_\alpha (t'-s)} {M_\alpha\Omega_\alpha} f'_\alpha[x_{t'}]f_\alpha[x_{s}],$](img1052.png)
![]() terms in
terms in
![]() , a fully quantum mechanical description of the bath.
, a fully quantum mechanical description of the bath.
![]() is positive definite and therefore, the term quadratic in
is positive definite and therefore, the term quadratic in ![]() in the original double path integral
exponentially suppresses strong deviations from the diagonal paths with
in the original double path integral
exponentially suppresses strong deviations from the diagonal paths with ![]() .
In this case, the expansion of the one-particle potential
.
In this case, the expansion of the one-particle potential ![]() ,
,