




Next: Feynman-Vernon Influence Functional Theories
Up: Unravelling and Decomposition into
Previous: Decomposition into Histories
Contents
Index
The decomposition of histories can now be simulated on a computer in order to actually
solve the Master equation. Here, we only describe the simplest version (spontaneous
emission, no driving field), starting from a
pure state
 of the total system. For more details, see Carmichael or Plenio/Knight.
of the total system. For more details, see Carmichael or Plenio/Knight.
Step 1: Fix a time step  . Calculate the probability
. Calculate the probability  of photon emission;
of photon emission;
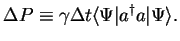 |
|
|
(147) |
Step 2: Compare  with a random number
with a random number

Step 3: Go back to Step 1.
This procedure (performed with small time-steps  up to a final time
up to a final time
 )
yields a `curve' of simulated states
)
yields a `curve' of simulated states
 ,
,
![$ t\in [0,t_{\rm final}]$](img664.png) in the
system Hilbert space
in the
system Hilbert space
 . The procedure is then repeated many times in order to
obtain time-dependent averages
. The procedure is then repeated many times in order to
obtain time-dependent averages
 of observables
of observables
 .
.
The entire procedure yields a density operator
 that solves the original Master equation,
Eq.(7.143): in one time step
that solves the original Master equation,
Eq.(7.143): in one time step  , we have
, we have
Remarks:
- The splitting of
 as
as
 is not unique, there
are ususally several ways of how to `unravel' the Master equation.
is not unique, there
are ususally several ways of how to `unravel' the Master equation.
- For more complicated Master equations, one has to extend and modify the above procedure.





Next: Feynman-Vernon Influence Functional Theories
Up: Unravelling and Decomposition into
Previous: Decomposition into Histories
Contents
Index
Tobias Brandes
2004-02-18
![]() . Calculate the probability
. Calculate the probability ![]() of photon emission;
of photon emission;
![]() with a random number
with a random number
![]()


![]() up to a final time
up to a final time
![]() )
yields a `curve' of simulated states
)
yields a `curve' of simulated states
![]() ,
,
![]() in the
system Hilbert space
in the
system Hilbert space
![]() . The procedure is then repeated many times in order to
obtain time-dependent averages
. The procedure is then repeated many times in order to
obtain time-dependent averages
![]() of observables
of observables
![]() .
.
![]() that solves the original Master equation,
Eq.(7.143): in one time step
that solves the original Master equation,
Eq.(7.143): in one time step ![]() , we have
, we have

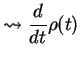
![$\displaystyle -i [H,\rho(t)]
- \kappa \Big\{ a^{\dagger} a {\rho} + \rho a^{\dagger} a - 2 a \rho a^{\dagger}
\Big\} .$](img671.png)