Electrostatics: multipole expansions

This is the simplest case: each of the 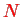$N$ subsystems (molecules) is considered as a distribution of charges with charge density $\rho_n({\bf x})$. The total charging energy is

$$E = \frac{1}{2}\sum_{nn'}\int \int d{\bf x}d{\bf x}' \frac{\rho_n({\bf x})\rho_{n'}({\bf x}')}{\vert{\bf x}-{\bf x}'\vert}.$$ (2.1)

We assume the molecules well separated with their center of mass at ${\bf R}_n$. We first consider the contribution from two different molecules, $n\ne n'$, and thus write

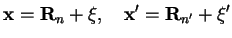$${\bf x} = {\bf R}_n + {\bf\xi},\quad {\bf x}' = {\bf R}_{n'} + {\bf\xi}'$$ (2.2)

and expand $\frac{1}{\vert{\bf x}-{\bf x}'\vert}$ for each pair $n,n'$, using the Taylor expansion

$$\frac{1}{\vert{\bf R}+{\bf a}\vert} = \frac{1}{R}\left(1 + \frac{{\bf a}^2+2{\bf R}{\bf a} }{R^2}\right)^{-1/2}$$ (2.3)

$$= \frac{1}{R}\left(1 - \frac{1}{2R^2}({\bf a}^2+ 2 {\bf R}{\bf a}) + \frac{3}{8R^4}({\bf a}^2+ 2 {\bf R}{\bf a})^2 + ...\right)$$

with ${\bf R}= {\bf R}_{nn'}\equiv {\bf R}_{n}-{\bf R}_{n'}$ and ${\bf a} = {\bf\xi}-{\bf\xi}'$. We assume uncharged molecules, i.e.

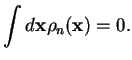$$\int d{\bf x} \rho_n({\bf x}) =0.$$ (2.4)

This yields

$$\int \int d{\bf x}d{\bf x}' \frac{\rho_n({\bf x})\rho_{n'}({\bf x}')}{\vert{\bf x}-{\bf x}'\vert} =$$

$$= \int \int d{\bf\xi}d{\bf\xi}'\rho_n({\bf\xi})\rho_{n'}({\bf\xi}') \frac{1}{R}\big(1 - \frac{1}{2R^2}([{\bf\xi-\xi'}]^2+ 2 {\bf R}[{\bf\xi-\xi'}])$$

$$+ \frac{3}{8R^4}([{\bf\xi-\xi'}]^2+ 2 {\bf R}[{\bf\xi-\xi'}])^2 + ...\big)$$

$$= \int \int d{\bf\xi}d{\bf\xi}'\rho_n({\bf\xi})\rho_{n'}({\bf\xi}')... ... \frac{3}{8R^4} 4 ({\bf R}[{\bf\xi-\xi'}] ) ({\bf R}[{\bf\xi-\xi'}] )+ ...\big)$$

$$= \int d{\bf\xi}d{\bf\xi}'\rho_n({\bf\xi})\rho_{n'}({\bf\xi}')\frac... ...^2}{\bf\xi \xi'} - \frac{3}{R^4} ({\bf R}{\bf\xi}) ({\bf R}{\bf\xi}') +...\big)$$

$$= \frac{{\bf d}_n {\bf d}_{n'}}{\vert{\bf R}_{nn'}\vert^3}-3 \frac{... ...rt{\bf R}_{nn'}\vert^5}+ ...,\quad {\bf R}_{nn'}\equiv {\bf R}_{n}-{\bf R}_{n'}$$ (2.5)

Here, the dipole moments are defined as

$${\bf d}_n = \int d{\bf x} {\bf x} \rho_n( {\bf x}),$$ (2.6)

and the remaining terms are dipole-quadrupole, quadrupole-quadrupole etc interaction energies which decay faster with increasing $R$. Usually the dipole-dipole interaction terms are dominant over the higher multipoles for the interaction between molecules.

Summarizing, we have

$$E = \frac{1}{2} \sum_n E_n^{\rm self} + \frac{1}{2} \sum_{nn'} \left( E_{nn'}^{\rm d-d} + E_{nn'}^{\mbox{\rm higher multipoles}}\right)$$ (2.7)

$$E_n^{\rm self} = \int \int d{\bf x}d{\bf x}' \frac{\rho_n({\bf x})\rho_n({\bf x}')}{\vert{\bf x}-{\bf x}'\vert}$$

$$E_{nn'}^{\rm d-d}({\bf R}_{nn'}) = \frac{{\bf d}_n {\bf d}_{n'}}{\vert{\bf R}_{nn'}\vert^3}-3 \frac{... ...{\vert{\bf R}_{nn'}\vert^5},\quad {\bf R}_{nn'}\equiv {\bf R}_{n}-{\bf R}_{n'}.$$

Exercise: Derive the expression for $E_{nn'}^{\rm d-d}$