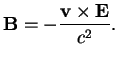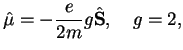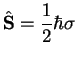Next: Spin-Orbit Coupling in Solids
Up: Spin-Orbit Coupling
Previous: Spin-Orbit Coupling
Contents
Index
In the hydrogen atom, the magnetic moment
 of the electron interacts with the magnetic field
of the electron interacts with the magnetic field
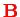 which the moving electron experiences in the electric field
which the moving electron experiences in the electric field
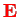 of the nucleus,
of the nucleus,
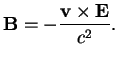 |
|
|
(3.8) |
One has
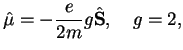 |
|
|
(3.9) |
where
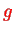 is the g-factor of the electron and
is the g-factor of the electron and
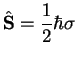 |
|
|
(3.10) |
is the electron spin operator. Therefore,
where
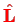 is the orbital angular momentum operator. This is reduced by an additional factor of
is the orbital angular momentum operator. This is reduced by an additional factor of
 (relativistic effects) such that
(relativistic effects) such that
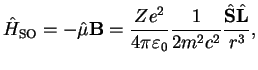 |
|
|
(3.12) |
which introduces a coupling term between spin and orbital angular momentum.
Note that Eq. (II.3.12) can directly been derived
by inserting Eq. (II.3.11) as
 into Eq. (II.3.6).
into Eq. (II.3.6).





Next: Spin-Orbit Coupling in Solids
Up: Spin-Orbit Coupling
Previous: Spin-Orbit Coupling
Contents
Index
Tobias Brandes
2005-04-26
![]() of the electron interacts with the magnetic field
of the electron interacts with the magnetic field
![]() which the moving electron experiences in the electric field
which the moving electron experiences in the electric field
![]() of the nucleus,
of the nucleus,