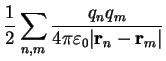Next: Coulomb Gauge
Up: Gauge invariance for many
Previous: Charge and current densities,
Contents
Index
This has to be worked out in detail, starting from the Lagrangian, which is described in Woolley [8]. The result is
Up to here everything is completely classical (just classical charges and fields). Quantization of the charge degrees of freedom is done canonically via
 .
.
Subsections





Next: Coulomb Gauge
Up: Gauge invariance for many
Previous: Charge and current densities,
Contents
Index
Tobias Brandes
2005-04-26
![$\displaystyle \frac{1}{2}\int d{\bf r} \left[ \varepsilon_0 \mathbf{E}_\perp^2 + \mu_0^{-1} \mathbf{B}^2\right]$](img1311.png)
![$\displaystyle \sum_n \frac{1}{2m_n} \left[ {\bf p}_n - q_n \mathbf{A}({\bf r}_n,t)\right] + V_{\rm Coul}+
V_{\bf EP}+V_{\bf gg}$](img1312.png)