Unsuccessful Attempt

Next: More Successful Attempt Up: Derivation Previous: Derivation Contents Index

Unsuccessful Attempt

A first guess to solve the stationary Schrödinger equation $\bgroup\color{col1}$ \mathcal{H}\Psi={\mathcal E}\Psi$\egroup$ for the total system would be a separation ansatz

$\displaystyle \mathcal{H}(q,p;X,P)\Psi(q,X)$	$\displaystyle =$	$\displaystyle {\mathcal E} \Psi(q,X)$
$\displaystyle \Psi(q,X)$	$\displaystyle =$	$\displaystyle \psi_e(q) \phi_n (X)$ unsuccessful $\displaystyle ,$	(2.1)

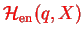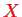which does not work because the interaction $\bgroup\color{col1}$ \mathcal{H}_{\rm en}(q,X)$\egroup$ depends on both $\bgroup\color{col1}$ q$\egroup$ and $\bgroup\color{col1}$ X$\egroup$ .

Tobias Brandes 2005-04-26