Math: Completeness, Dirac notation

Next: Operators and The Two-Level-System Up: Axioms of Quantum Mechanics Previous: First Axiom: States as Contents

Math: Completeness, Dirac notation

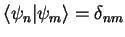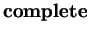Def.: An orthonormal basis $\{\psi_n\}$ , $\langle \psi_n \vert \psi_m \rangle =\delta_{nm}$ , of a Hilbert space is called ${\bf complete}$ if no vector 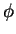$\phi$ (except the zero vector) is orthogonal to all $\psi_n$ .

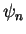In the following, we will mostly deal with Hilbert spaces that have a complete orthonormal basis, which guarantees the expansion of any wave function into a linear combination of basis vectors. In most cases, the orthonormal basis consists of the eigenfunctions (eigenvectors) of the Hamilton operator $\hat{H}$ .

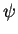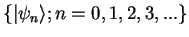In quantum mechanics it has become common to use the symbol (`ket') $\vert\psi\rangle$ for a wave function (Hilbert space vector) instead of $\psi$ . The expansion of an arbitrary ket $\vert\psi\rangle$ into the orthonormal basis $\{ \vert\psi_n\rangle; n=0,1,2,3,... \}$ then can be written as

$\displaystyle \vert\psi\rangle$

$\displaystyle =$

$\displaystyle \sum_{n=0}^{\infty} \vert\psi_n\rangle \langle\psi_n \vert\psi\rangle .$

(177)

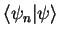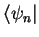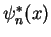Here, the scalar product, that is the `bracket' $\langle\psi_n \vert\psi\rangle$ , gives rise to define the bra vector (from `bra -cket') $\langle \psi_n \vert$ as the state with wave function $\psi^*_n(x)$ . This means that

$\displaystyle \langle \psi \vert$

$\displaystyle =$

$\displaystyle \sum_{n=0}^{\infty} \langle \psi_n \vert \langle\psi \vert\psi_n \rangle .$

(178)

A very convenient way to memorize and use the completeness property is the `insertion of the 1',

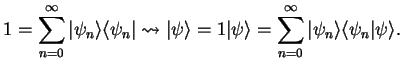$\displaystyle 1 = \sum_{n=0}^{\infty} \vert\psi_n\rangle \langle\psi_n \vert \l... ...rangle= \sum_{n=0}^{\infty} \vert\psi_n\rangle \langle\psi_n \vert\psi\rangle .$

(179)

Next: Operators and The Two-Level-System Up: Axioms of Quantum Mechanics Previous: First Axiom: States as Contents

Tobias Brandes 2004-02-04