




Next: Derivation of Master equation
Up: Thermal Bath Correlation Function
Previous: Properties of
Contents
Index
With explicit expressions like Eq. (7.59) and Eq. (7.61),
one can now directly assess the validity of the Markov assumption (Assumption 2a above):
` the bath correlation function
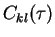 is strongly
peaked around
is strongly
peaked around 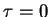 with a peak width
with a peak width
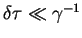 , where
, where
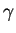 is a typical rate of change of
is a typical rate of change of
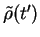 .' For example,
for
.' For example,
for  ,
,
 ,
and within the model
,
and within the model
 , Eq.(7.54),
one has
, Eq.(7.54),
one has
 , cf. Eq.(7.61).
This would mean
, cf. Eq.(7.61).
This would mean
which is fulfilled for large  (
(
 ),
),  ,
and small
,
and small  .
The condition of small
.
The condition of small  is consistent with the Born approximation (perturbation theory
in the coupling to the bath).
is consistent with the Born approximation (perturbation theory
in the coupling to the bath).
Tobias Brandes
2004-02-18