




Next: Exact solution
Up: A `Mini-Molecule': Perturbation Theory
Previous: A `Mini-Molecule': Perturbation Theory
Contents
Index
Figure:
Vector representation of left and right lowest states of double well potential.
|
|
The two-level system describes a particle in an `abstract' double well with just two states. We associate a Hamiltonian
 with the two isolated wells: the unperturbated Hamiltonian is a two-by-two matrix,
with the two isolated wells: the unperturbated Hamiltonian is a two-by-two matrix,
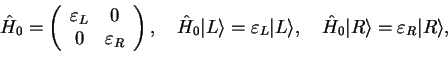 |
|
|
(2.2) |
i.e.,
 is eigenvector of
is eigenvector of
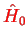 with eigenvalue
with eigenvalue
 and
and
 is eigenvector with eigenvalue
is eigenvector with eigenvalue
 . The tunnel effect is considered as a perturbation
. The tunnel effect is considered as a perturbation
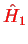 to
to
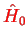 ,
,
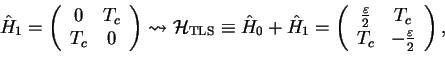 |
|
|
(2.3) |
with a tunnel coupling
 (real parameter). We furthermore set
(real parameter). We furthermore set
 and
and
 .
.
Subsections





Next: Exact solution
Up: A `Mini-Molecule': Perturbation Theory
Previous: A `Mini-Molecule': Perturbation Theory
Contents
Index
Tobias Brandes
2005-04-26
![\includegraphics[width=0.4\textwidth]{dwell3b}](img112.png)
![\includegraphics[width=0.4\textwidth]{dwell3a}](img113.png)
![]() with the two isolated wells: the unperturbated Hamiltonian is a two-by-two matrix,
with the two isolated wells: the unperturbated Hamiltonian is a two-by-two matrix,