




Next: Adiabaticity and Geometric Phases
Up: The Born-Oppenheimer Approximation
Previous: More Successful Attempt
Contents
Index
We now have to justify the neglect of the underlined term in
Up to here, everything was still fairly general. Now we make out choice for
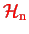 as just the kinetic energy of the nuclei,
as just the kinetic energy of the nuclei,
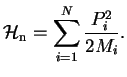 |
|
|
(2.9) |
We simplify the following discussion by writing
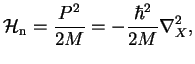 |
|
|
(2.10) |
which refers to a) a single relative motion of two nuclei of effective mass
 , or alternatively b) represents an `abstract notation' for
, or alternatively b) represents an `abstract notation' for
 (to which the following transformations can easily be generalised).
(to which the following transformations can easily be generalised).
We write
This term is therefore determined by the derivative of the electronic part with respect to the nuclear positions
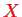 , and it has the factor
, and it has the factor
 in front. The `handwaving' argument now is to say that the derivatives
in front. The `handwaving' argument now is to say that the derivatives
 and
and
 are small.
are small.





Next: Adiabaticity and Geometric Phases
Up: The Born-Oppenheimer Approximation
Previous: More Successful Attempt
Contents
Index
Tobias Brandes
2005-04-26
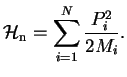
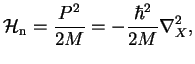
![$\displaystyle \mathcal{H}_{\rm n}\psi_e \phi_n - \psi_e\mathcal{H}_{\rm n} \phi...
... \left[\nabla_X^2 \psi_e(q,X) \phi_n(X) - \psi_e(q,X)\nabla_X^2\phi_n(X)\right]$](img781.png)
![$\displaystyle -\frac{\hbar^2}{2M} \Big[ \nabla_X \left\{\phi_n \nabla_X\psi_e + \phi_e \nabla_X\psi_n \right\}
-\psi_e\nabla_X^2\phi_n\Big]$](img782.png)
![$\displaystyle -\frac{\hbar^2}{2M} \Big[ 2 \nabla_X\phi_n \nabla_X\psi_e
+ \phi_n \nabla_X^2 \psi_e\Big].$](img783.png)