Adiabaticity and Geometric Phases

The electronic part equation

$$\left[\mathcal{H}_{\rm e}(q,p)+\mathcal{H}_{\rm en}(q,X)\right] \psi_e(q,X) = E(X) \psi_e(q,X)$$ (2.12)

usually should give not only one but a whole set of eigenstates,

$$\left[\mathcal{H}_{\rm e}+\mathcal{H}_{\rm en}\right] \vert\alpha(X)\rangle = E_\alpha(X)\vert\alpha(X)\rangle.$$ (2.13)

Assume that for a fixed 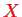$X$ we have orthogonal basis of the electronic Hilbert space with states $\vert\alpha(X)\rangle$, no degeneracies and a discrete spectrum $E_\alpha(X)$,

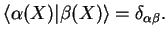$$\langle \alpha(X)\vert \beta(X)\rangle = \delta_{\alpha\beta}.$$ (2.14)

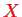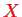Adiabaticity means that when $X$ is changed slowly from $X\to X'$, the corresponding state slowly changes from $\vert\alpha(X)\rangle\to\vert\alpha(X')\rangle$ and does not jump to another $\alpha'\ne \alpha$ like $\vert\alpha(X)\rangle\to\vert\alpha'(X')\rangle$. In that case, we can use the $\vert\alpha(X)\rangle$ as a basis for all $X$ and write

$$\Psi(q,X)=\sum_\alpha \phi_\alpha (X) \psi_\alpha(q,X).$$ (2.15)

Now

$$\mathcal{H}\sum_\alpha \vert\phi_\alpha\rangle_n \otimes \vert\psi_\alpha\rangle_e = \sum_\alpha \left[ \mathcal{H}_{\rm n} + E_\alpha(X)\right] \vert\phi_\alpha\rangle_n \otimes \vert\psi_\alpha\rangle_e,$$ (2.16)

and taking the scalar product with a $\langle \psi_\alpha\vert$ of the Schrödinger equation $\mathcal{H} \Psi=\mathcal{E} \Psi$ therefore gives

$$\left[\langle \psi_\alpha\vert \mathcal{H}_{\rm n}\vert\psi_\alph... ...pha(X)\right] \vert\phi_\alpha\rangle_n = \mathcal{E} \vert\phi_\alpha\rangle_n$$ (2.17)

This is the Schrödinger equation for the nuclei within the adiabatic approximation. Now using again

$$\mathcal{H}_{\rm n} = -\frac{\hbar^2}{2M}\nabla_X^2 \leadsto \mathcal{H}_{\rm n} \psi_\alpha(q,X) \phi_\alpha(X)$$

$$= -\frac{\hbar^2}{2M}\Big[\psi_\alpha(q,X) \nabla^2_X \phi_\alpha(X) + \phi_\alpha(X) \nabla^2_X \psi_\alpha(q,X)$$

$$+ 2 \nabla_X \phi_\alpha(X) \nabla_X \psi_\alpha(q,X)\Big]$$ (2.18)

and therefore the nuclear Schrödinger equation becomes

$$\left[\langle \psi_\alpha\vert \mathcal{H}_{\rm n}\vert\psi_\alph... ...ght] \vert\phi_\alpha\rangle_n = \mathcal{E} \vert\phi_\alpha\rangle_n \leadsto$$

$$\left[-\frac{\hbar^2}{2M}\nabla_X^2 +E_\alpha(X) - \langle \psi_\... ...\nabla_X}{M}\vert \psi_\alpha\rangle \nabla_X \right] \vert\phi_\alpha\rangle_n$$

$$= \mathcal{E} \vert\phi_\alpha\rangle_n$$ (2.19)

which can be re-written as

$$\left[-\frac{\hbar^2}{2M}\nabla_X^2 +E_\alpha(X) -\frac{\hbar^2}{2M}G(X) -\frac{\hbar^2}{M}F(X)\nabla_X \right] \vert\phi_\alpha\rangle_n = \mathcal{E} \vert\phi_\alpha\rangle_n$$

$$G(X)\equiv \langle \psi_\alpha\vert\nabla^2_X\psi_\alpha\rangle,\quad F(X)\equiv \langle \psi_\alpha\vert\nabla_X\psi_\alpha\rangle ,$$ (2.20)

where we followed the notation by Mead and Truhlar in their paper J. Chem. Phys. 70, 2284 (1979). Eq. (E.5.2) is an important result as it shows that the adiabatic assumption leads to extra terms $F(X)$ and $G(X)$ in the nuclear Schrödinger equation in BO approximation on top of just the potential created by the electrons. In particular, the term $F(X)$ is important as it leads to a non-trivial geometrical phase in cases where the curl of $F(X)$ is non-zero. This has consequences for molecular spectra, too. geometric phases such as the abelian Berry phase and the non-abelian Wilczek-Zee holonomies play an important role in other areas of modern physics, too, one example being `geometrical quantum computing'. For more info on the geometric phase in molecular systems, cf. the Review by C. A. Mead, Prev. Mod. Phys. 64, 51 (1992).