



Next: ** A more general
Up: The Tunnel Effect and
Previous: Transmission coefficient (10 min)
Contents
Consider the case
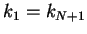 in (2.14).
Use the definitions for
in (2.14).
Use the definitions for 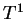 (
(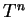 correspondingly) and
correspondingly) and 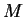
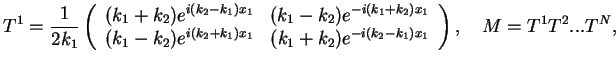 |
(20) |
to show that the determinant of the transfer matrix 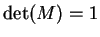 .
.
Tobias Brandes
2004-02-04