



Next: Harmonic Oscillator: Parity
Up: The Hilbert space of
Previous: Infinite Well Hilbert Space
Contents
For the harmonic oscillator, the situation is completely analogous. The difference now
is that the potential is no longer the infinite well but a harmonic potential.
The wave functions are not defined on the finite interval ![$ [0,L]$](img379.png) but on the infinite
interval
but on the infinite
interval
![$ [-\infty,\infty]$](img1092.png) .
.
The eigen functions 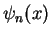 of the harmonic oscillator form the basis
of a linear vector space
of the harmonic oscillator form the basis
of a linear vector space
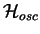 of functions
of functions 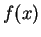 defined on the
interval
defined on the
interval
![$ [-\infty,\infty]$](img1092.png) with
with
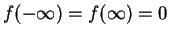 .
The
.
The 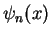 form an orthonormal basis:
form an orthonormal basis:
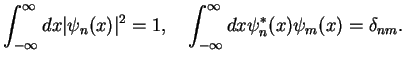 |
|
|
(251) |
(We can omit the 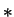 here because the
here because the 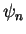 are real).
Note that the orthonormal basis is of infinite dimension because there are infinitely many
are real).
Note that the orthonormal basis is of infinite dimension because there are infinitely many
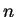 . The infinite dimension of the vector space (function space)
. The infinite dimension of the vector space (function space)
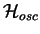 is the main difference to ordinary, finite dimensional vector spaces
like the
is the main difference to ordinary, finite dimensional vector spaces
like the 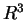 .
Any function
.
Any function
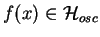 (like any arbitrary vector in, e.g., the vector space
(like any arbitrary vector in, e.g., the vector space 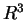 ) can be expanded into
a linear combination of basis `vectors', i.e. eigen functions
) can be expanded into
a linear combination of basis `vectors', i.e. eigen functions 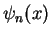 :
:
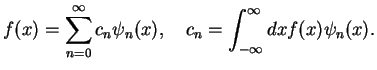 |
|
|
(252) |
We start to count the eigenstates from 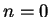 and not
and not  as for the infinite well.
To prove the orthogonality (4.27) is a bit more difficult for the
harmonic oscillator then for the infinite potential well. It can be done by using the properties of the
Hermite polynomials.
as for the infinite well.
To prove the orthogonality (4.27) is a bit more difficult for the
harmonic oscillator then for the infinite potential well. It can be done by using the properties of the
Hermite polynomials.




Next: Harmonic Oscillator: Parity
Up: The Hilbert space of
Previous: Infinite Well Hilbert Space
Contents
Tobias Brandes
2004-02-04
![]() of the harmonic oscillator form the basis
of a linear vector space
of the harmonic oscillator form the basis
of a linear vector space
![]() of functions
of functions ![]() defined on the
interval
defined on the
interval
![]() with
with
![]() .
The
.
The ![]() form an orthonormal basis:
form an orthonormal basis: